7.6 Empirical Data
One might argue that our conclusions are based on asymptotic expansions only, thus it is not certain if the observations hold for practical relevant settings. To overcome this weak point, we obtained numerical results that are provided in this section.
We start providing some results concerning the phase transition and the “critical” value ε = 0, for ordinary and symmetric bipartite graphs. Recall that the latter value corresponds to the relation m = n, that is the number of edges equals half the number of nodes. From Theorem 7.2.1, we conclude that the probability that no complex component occurs drops from ![]() to
to ![]() . The numerical results given in Table 7.2 exhibit a similar behavior, see also Tables 7.4 and 7.5. Note that the observed number of complex components is slightly below the expectation calculated using the asymptotic approximation. However, the accuracy increases as the number of nodes increases.
. The numerical results given in Table 7.2 exhibit a similar behavior, see also Tables 7.4 and 7.5. Note that the observed number of complex components is slightly below the expectation calculated using the asymptotic approximation. However, the accuracy increases as the number of nodes increases.
Table 7.2 Number of Graphs Containing a Complex Component out of 5 × 105 Graphs Generated for Each Setup.
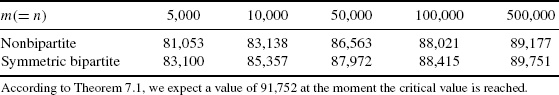
Table 7.4 The Excess of the Complex Part of a Symmetric Bipartite Graph Possessing m Nodes of Each Type and n = (1 − ε)m Keys.
Table 7.5 The Excess of the Complex ...
Get Statistical and Machine Learning Approaches for Network Analysis now with the O’Reilly learning platform.
O’Reilly members experience books, live events, courses curated by job role, and more from O’Reilly and nearly 200 top publishers.

