Student Solutions Manual to Accompany Loss Models: From Data to Decisions, Fourth Edition

CHAPTER 9

CHAPTER 9 SOLUTIONS

9.1   SECTION 9.1

9.1 The number of claims, N, has a binomial distribution with m = number of policies and q = 0.1. The claim amount variables, X₁, X₂,…, all are discrete with Pr(X_j = 5,000) = 1 for all j.

9.2 (a) An individual model is best because each insured has a unique distribution. (b) A collective model is best because each malpractice claim has the same distribution and there are a random number of such claims. (c) Each family can be modeled with a collective model using a compound frequency distribution. There is a distribution for the number of family members and then each family member has a random number of claims.

9.2   SECTION 9.2

9.3

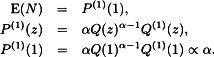
9.4 The Poisson and all compound distributions with a Poisson primary distribution have a pgf of the form P(z) = exp{λ[P₂(z) − 1]} = [Q(z)]^λ, where Q(z) = exp[P₂(z) − 1].

The negative binomial and geometric distributions and all compound distributions with a negative binomial or geometric primary distribution have P(z) = {1 − β[P₂(z) − 1]}^−r = [Q(z)]^r, where Q(z) = {1 − β[P₂(z) − 1]}⁻¹.

The same is true for the binomial distribution and binomial-X compound distributions with α = m and Q(z) = 1 + q[P₂(z) − 1].

The zero-truncated and zero-modified distributions cannot be written in this form.

9.3   SECTION 9.3

9.5 To simplify writing the expressions, let

and similarly for S. For the first moment, , and ...