Topological Optimization and Optimal Transport

Indeed, we can write in the 2-marginals case, det(x₁, x₂) = 〈x₁, Rx₂〉, where R: ℝ₂ → ℝ₂ is the rotation of angle −π/2. Hence, since μ₁ and μ₂ have finite second moments, up to a change of variable x̃₂ = Rx₂, the problem (_Det) in (5.1) is equivalent to the classical Brenier’s optimal transportation problem:

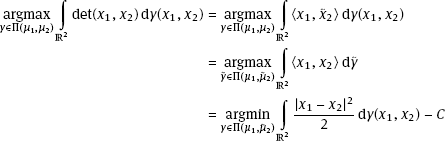
where

In the sequel, we are going to construct maximizers for (5.1), thanks to some properties of the Kantorovich potentials of the dual problem ...