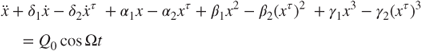Chapter 6Time-Delayed Nonlinear Oscillators
In this chapter, analytical solutions for period-m motions in a time-delayed, nonlinear oscillator will be presented through the Fourier series, and the stability and bifurcation analyses of the corresponding periodic motions are presented through the eigenvalue analysis. Analytical bifurcation trees of periodic motions to chaos will be presented through the frequency-amplitude curves. Trajectories and amplitude spectrums of periodic motions in such a time-delayed nonlinear system are illustrated numerically for a better understanding of time-delayed nonlinear dynamical systems.
6.1 Analytical Solutions
In this section, the analytical solutions of periodic motions in time-delayed, nonlinear systems will be developed through finite Fourier series. Consider a periodically forced, time-delayed, nonlinear oscillator as
where 
and 
. Coefficients in Equation (6.1) are 
and 
for linear damping, and for linear springs and delay, and for quadratic ...
Get Toward Analytical Chaos in Nonlinear Systems now with the O’Reilly learning platform.
O’Reilly members experience books, live events, courses curated by job role, and more from O’Reilly and nearly 200 top publishers.
