Tunable Lasers Handbook

8 Tunable External-Cavity Semiconductor Lasers 37S

media or by going to tapered-stripe gain media. Recently, up to -1W output has

been obtained from an external cavity laser with a tapered-stripe gain medium

[43]. The output beam was described as diffraction-limited. The coupling effi-

ciency to optical fiber was not reported.

5. FEEDBACK MODEL

5.1 Effective Reflectance

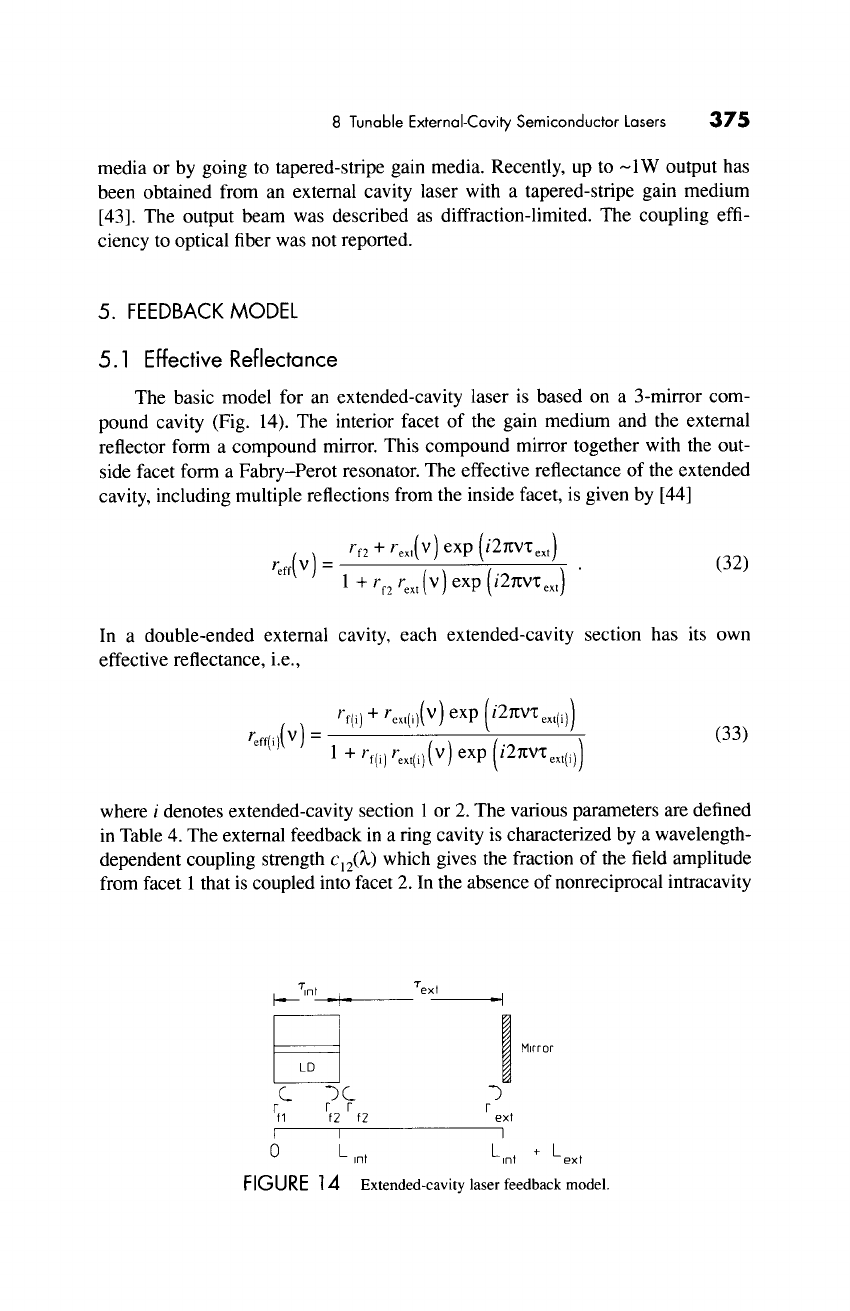
The basic model for an extended-cavity laser is based on a 3-mirror com-

pound cavity (Fig. 14). The interior facet of the gain medium and the external

reflector form a compound mirror. This compound mirror together with the out-

side facet form a Fabry-Perot resonator. The effective reflectance of the extended

cavity, including multiple reflections from the inside facet, is given by [44]

rf2 + rext(V) exp (i2%'VX ext)

reef(V) = 1 + rf2 rex t (v)

exp (i2gV~ext) " (32)

In a double-ended external cavity, each extended-cavity section has its own

effective reflectance, i.e.,

rf(i) + rext(i)(v) exp (i2~VT'ext(i))

Feff(i)(V) = 1 "+" Kf(i)Fext(i)(V ) exp

(i2~V'l;ext(,))

(33)

where i denotes extended-cavity section 1 or 2. The various parameters are defined

in Table 4. The external feedback in a ring cavity is characterized by a wavelength-

dependent coupling strength C l20~) which gives the fraction of the field amplitude

from facet 1 that is coupled into facet 2. In the absence of nonreciprocal intracavity

i Tint ~_ Text

I ~ "IIDt

Lol

1

C bC "3

r r r r

fl f2 f2 ext

I I I

O L L +L

int ~nt ext

FIGURE 14

Extended-cavity laser feedback model.

376 Paul Zorabedian

elements such as an optical isolator, the coupling between the two facets obeys

reciprocity, i.e., c12(~) = c21(~). Multiple reflections between the facets and the

external mirrors defining the ring can be neglected because there are no (inten-

tional) standing waves in the cavity. However, sometimes spurious etalons exist

between the residual reflections of the facets and the intracavity optics.

5.2 Threshold Current

The gain coefficient per unit length is given by

g(',~,) = 711- Itr(~,)] , (34)

where "t is a constant independent of ~, I is the pump current, and Itr(~) is the

transparency current. Note that the previously-defined confinement factor F has

been lumped in with the constant % The threshold magnitude condition states

that the round-trip gain equals the total round-trip loss. This leads to the follow-

ing general expression for the threshold current:

Ith "- ~[0~ int -I- O~ mir] -I- Itr , (35)

where

O~mi r

is represents the mirror loss for the appropriate cavity configuration.

These are given below.

5.3 Mirror

Losses

The mirror loss in an extended-cavity configuration is given by

1'hi 1 /

mir-- Lint rf,roff( )

(36)

TABLE 4 External Feedback Model Parameters

rfl, rf2

v

rextl (v), rext2(v)

6'

"l;ext 1 -" c/2Lext 1

"l;ext2 = c/2Lext2

Amplitude reflectances of facets

Optical frequency

Amplitude reflectances of extended cavity sections (lumping, coupling, filter,

and mirror losses)

Speed of light

Round-trip time of extended cavity section with length Lextl

Round-trip time of extended cavity section with length Lext2