2
Probabilistic Approaches for Treating Uncertainty
Probability has been, and continues to be, a fundamental concept in the context of risk assessment. Unlike the other measures of uncertainty considered in this book, probability is a single-valued measure.
The common axiomatic basis of probability is found in Kolmogorov's (1933) probability axioms. Let 
denote events in a sample space 
. The following probability axioms are assumed to hold:
(2.1)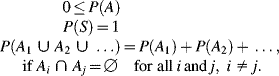 
These axioms specify that probability is a non-negative, normalized, and additive measure.
Different types of operational or conceptual interpretations of probability can be distinguished. Among the most common are:
- Classical
- Relative frequency
- Subjective
- Logical.
Modern probability theory is not based on a particular interpretation of probability, although its standard language is best suited to the classical and relative frequency interpretations (Aven, 2012). In the context of risk assessment, the relative frequency and subjective interpretations are most common. Relative frequency probability is broadly recognized as the proper representation of variation in large populations (aleatory uncertainty), also among proponents of alternative ...
