December 2020
Intermediate to advanced
1064 pages
49h 13m
English
Content preview from Advanced Engineering Mathematics, 7th Edition
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.
5 Chapter in Review Answers to selected odd-numbered problems begin on page ANS-14.
In Problems 1 and 2, answer true or false without referring back to the text.
- The general solution of x2 y″ + xy′ + (x2 − 1)y = 0 is y = c1J1(x) + c2J−1(x). _______
- Since x = 0 is an irregular singular point of x3y″ − xy′ + y = 0, the DE possesses no solution that is analytic at x = 0. _______
- Both power series solutions of y″ + ln(x + 1)y′ + y = 0 centered at the ordinary point x = 0 are guaranteed to converge for all x in which one of the following intervals?
(a) (– ∞, ∞)
(b) (–1, ∞)
(c) [–
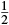, ](d) [–1, 1]
- x = 0 is an ordinary point of a certain linear differential ...
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
and much more.
Read now
Unlock full access
More than 5,000 organizations count on O’Reilly
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.Julian F.
I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.Addison B.
I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.Amir M.
I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.