October 2007
Intermediate to advanced
780 pages
21h 16m
English
Content preview from Analysis of Multiconductor Transmission Lines, 2nd Edition
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.
2
THE TRANSMISSION-LINE EQUATIONS FOR TWO-CONDUCTOR LINES
In this chapter, we will show the derivation of the transmission-line equations for two-conductor lines from two points of view. First, they will be derived from the integral form of Maxwell's equations, and second they will be derived from the per-unit-length distributed parameter equivalent circuit. Both methods allow the incorporation of conductor losses. In Chapter 3, we will repeat this for a general (n + 1)-conductor multiconductor transmission line (MTL). The process will be identical to that for a two-conductor line, but the details will be a bit more tedious. Nevertheless, the transmission-line equations for an MTL, written using matrix notation, will be identical in form to those for a two-conductor line.
2.1 DERIVATION OF THE TRANSMISSION-LINE EQUATIONS FROM THE INTEGRAL FORM OF MAXWELL'S EQUATIONS
Recall Faraday's law in integral form [A.1,A.6]:
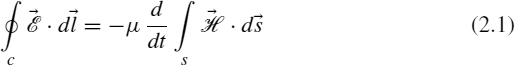
This is illustrated in Figure 2.1. The differential path length around the closed contour c that bounds the open surface s is denoted by 
. A differential surface area of surface s is denoted by 
, where is a unit normal to the surface. The direction of the contour c and ...
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
and much more.
Read now
Unlock full access
More than 5,000 organizations count on O’Reilly
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.Julian F.
I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.Addison B.
I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.Amir M.
I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.