Problem 8
Jacob Bernoulli and His Golden Theorem (1713)
Problem. Show that the probability of an equal number of heads and tails when a fair coin is tossed 2m times is approximately 
for large m.
Solution. Let X be the number of heads for 2m independent tosses of a fair coin. Then X 
B(2m, 1/2) and
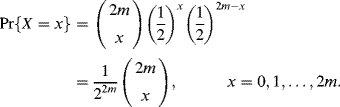
The probability of an equal number of heads and tails is
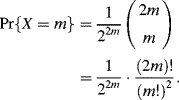
Applying Stirling's formula 
for large N, we have
(8.1)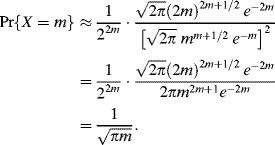 
8.1 Discussion
The last formula implies that, for a fair coin, the probability of an equal number of heads and tails is inversely proportional to 
. Table 8.1 shows that, as more coins are tossed, it becomes less likely that there will be an equal number of heads and tails.
Table 8.1 The Probability of an Equal Number of Heads and Tails When a Fair Coin is Tossed 2m Times.
| Number ... |
