Credit Models and the Crisis: A Journey into CDOs, Copulas, Correlations and Dynamic Models

4

Consistency across Capital Structure: Implied Copula

In the Implied Copula approach, a factor copula structure is assumed, similar to the one-factor Gaussian Copula approach seen earlier. However, this time we do not model the copula explicitly, but we model default probabilities conditional on the systemic factor S of the copula: the copula will then be “hidden” inside these conditional probabilities that will be calibrated to the market. Hence the name “Implied” Copula. In illustrating the Implied Copula we will also assume a large pool homogeneous model in that the default probabilities of single names will all be taken equal to each other.

Let us consider, for simplicity, survival probabilities that are associated to a constant-in-time hazard rate. We know that if we have a constant-in-time (possibly random) hazard rate λ for name i, then the survival probability is

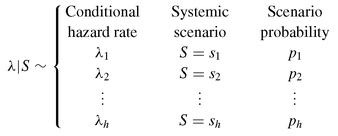
The Implied Copula approach postulates the following “scenario” distribution for the hazard rate λ conditional on the systemic factor S:

This way the default probability for each single name i = 1, . . . , M is, conditional on the systemic factor S,

Compared with the Gaussian factor copula case:

Unconditionally, ...