Derivatives Models on Models

6

Knock-in/Out Margrabe^*

with Jørgen Haug

In this chapter we push the Black-Scholes-Merton (BSM) formula to the limit by using it to value exchange-one-asset-for-another options with knock-in or knock-out provisions that depend on the ratio of the two asset prices. These option contracts are relevant to investors and traders concerned with the relative performance of securities, and they crop up in M&As.

1 Margrabe Options

Exchange-one-asset-for-another options give the owner the right to exchange asset S₂ for asset S₁ at expiration and were originally analyzed by Margrabe (1978).¹ The payoff from such a call option is

where (·)⁺ is the positive part. Margrabe considered the special case when the two asset prices follow geometric Brownian motion:

where μ_i and σ_i are the instantaneous return and volatility of asset i = 1, 2, and dz₁ and dz₂ are correlated Wiener processes with correlation coefficient ρ. The price of a call option with τ years left to maturity, derived in the appendix as a prelude to extensions with barriers, is then

where

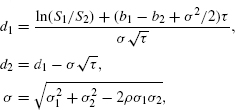
and b_i is the cost of carry of asset i = 1, ...