Design Theory, 2nd Edition

1

Steiner Triple Systems

 

1.1 The existence problem

A Steiner triple system is an ordered pair (S, T), where S is a finite set of points or symbols, and T is a set of 3-element subsets of S called triples, such that each pair of distinct elements of S occurs together in exactly one triple of T. The order of a Steiner triple system (S, T) is the size of the set S, denoted by |S|.

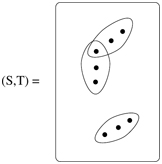
Figure 1.1: Steiner triple system.

Example 1.1.1 (a) S = {1},  T = φ

(b) S = {1, 2, 3 },  T = {{1, 2, 3}}

(c) S = {1, 2, 3, 4, 5, 6, 7 },  T = {{1, 2, 4 }, {2, 3, 5}, {3, 4, 6}, {4, 5, 7}, {5, 6, 1}, {6, 7, 2}, {7, 1, 3}}

(d) S = {1, 2, 3, 4, 5, 6, 7, 8, 9 } ...