Digital Alias-free Signal Processing

14

Signal Transforms

The idea of exploiting sampling randomization to achieve the capability of alias-free signal processing in a wide frequency range is attractive and often attracts attention. However, to achieve good results processing of nonuniformly represented signals must be carried out using special algorithms that take into account the specifics of this sampling approach. While this may seem to be obvious, it is less clear what criteria are required for evaluating the degree of matching the specifics of signal sampling and processing. This is not a trivial question. The following discussions explain to some extent why this is so. More about this is discussed in Chapter 18.

14.1 Problem of Matching Signal Processing to Sampling

These discussions will start by considering an example. Suppose that the mean power P_x of a wideband signal x(t) component at frequency ω_i that exceeds the mean sampling frequency ω_s has to be estimated. The application of random sampling is clearly indicated. At first glance it seems that this task can be solved by estimating the Fourier coefficients a_i and b_i at the frequency ω_i on the basis of the often applied formulae

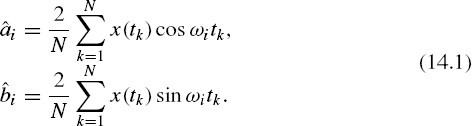
The required estimate is then given as

Although these equations look like their conventional counterparts, they are in fact modified ...