Discrete Stochastic Processes and Optimal Filtering

6.9. Example of the application of the LMS algorithm

Let us recall the modelization of an AR process.

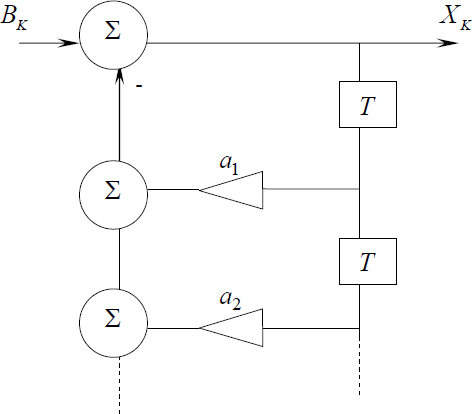
Thus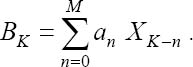

By multiplying the two members by X_K−l and by taking the expectations, it becomes:

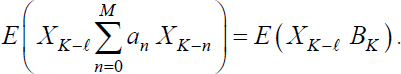
If then

As B_K is a white noise and unique B_K is dependent on X_K.

Thus, by stating:

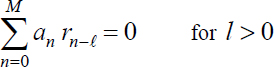
and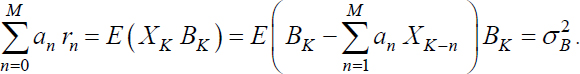

By noting a₀ = 1 and by using the matrix expression, this becomes:

For an AR process of order 1, let the following AR process be X_K = −a X_K−1 + B_K where B_K is a centered white noise of variance .

The problem consists ...