Engineering Mathematics, Volume I, Second Edition

3

Curvature

Let P be any point on a given curve and Q a neighboring point of P such that the arc PQ is concave towards its chord. Let the normals at P and Q intersect at N.

 

 

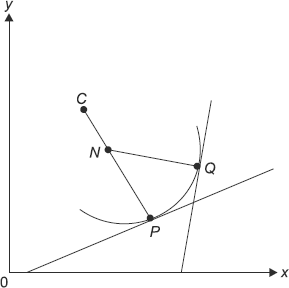
When Q → P, N tends to a definite position C, called the center of curvature of the curve at P. The distance CP is called the radius of curvature of the curve at the point P and is denoted by ρ. The circle with center at C and the radius ρ, equal to CP, is called the circle of curvature of the given curve at the point P. Any chord of the circle of curvature drawn through the point P is called the chord of curvature. The reciprocal of the radius of curvature is called ...