book

Engineering Mathematics

Name: Engineering Mathematics
Author: Babu Ram
ISBN: 9788131726914

by Babu Ram

June 2009

Intermediate to advanced

1100 pages

42h 22m

English

Pearson India

Read now

Unlock full access

Cover
Title Page
Contents
Dedication
Preface
Symbols and Basic Formulae
Part I
1. Sequences and Series
1.1 Sequences1.2 Convergence of Sequences1.3 The Upper and Lower Limits of a Sequence1.4 Cauchy's Principle of Convergence1.5 Monotonic Sequence1.6 Theorems on Limits1.7 Subsequence1.8 Series1.9 Comparison Tests1.10 D’ Alembert's Ratio Test1.11 Cauchy's Root Test1.12 Raabe's Test1.13 Logarithmic Test1.14 De Morgan – Bertrand Test1.15 Gauss's Test1.16 Cauchy's Integral Test1.17 Cauchy's Condensation Test1.18 Kummer's Test
1.19 Alternating Series
1.20 Absolute Convergence of a Series1.21 Convergence of the Series of the Type1.22 Derangement of Series1.23 Nature of Non-absolutely Convergent Series1.24 Effect of Derangement of Non-absolutely Convergent Series1.25 Uniform Convergence1.26 Uniform Convergence of a Series of Functions1.27 Properties of Uniformly Convergent SeriesExercises
2. Mean Value Theorems and Expansion of Functions
2.1 Leibnitz's Theorem and Its Applications2.2 General Theorems2.3 Taylor's Infinite Series and Power Series Expansion2.4 Maclaurin's Infinite Series2.5 Expansion of Functions2.6 Indeterminate formsExercises

3. Curvature
3.1 Radius of Curvature of Intrinsic Curves3.2 Radius of Curvature for Cartesian Curves3.3 Radius of Curvature for Parametric Curves3.4 Radius of Curvature for Pedal Curves3.5 Radius of Curvature for Polar Curves3.6 Radius of Curvature at the Origin3.7 Centre of Curvature3.8 Evolutes and Involutes3.9 Equation of the Circle of Curvature3.10 Chords of Curvature Parallel to the Coordinate Axes3.11 Chord of Curvature in Polar CoordinatesExercises
4. Asymptotes and Curve Tracing
4.1 Determination of Asymptotes When the Equation of the Curve in Cartesian form is given4.2 The Asymptotes of the General Rational Algebraic Curve4.3 Asymptotes parallel to the Coordinate Axes4.4 Working Rule for Finding Asymptotes of Rational Algebraic Curve4.5 Intersection of a Curve and its Asymptotes4.6 Asymptotes by Expansion4.7 Asymptotes of the Polar Curves4.8 Circular Asymptotes4.9 Curve Tracing (Cartesian Equations)4.10 Curve Tracing (Polar Equations)4.11 Curve Tracing (Parametric Equations)Exercises
5. Partial Differentiation
5.1 Continuity of a Function of Two Variables5.2 Differentiability of a Function of Two Variables5.3 The Differential Coefficients5.4 Distinction Between Derivatives and Differential Coefficients5.5 Higher-Order Partial Derivatives5.6 Envelopes and Evolutes5.7 Homogeneous Functions and Euler's Theorem5.8 Differentiation of Composite Functions5.9 Transformation From Cartesian to Polar Coordinates and Vice Versa5.10 Taylor's Theorem For Functions of Several Variables5.11 Extreme Values5.12 Lagrange's Method of Undetermined Multipliers5.13 Jacobians5.14 Properties of Jacobian5.15 Necessary and Sufficient Conditions for Jacobian to Vanish5.16 Differentiation Under the Integral SignExercises
6. Beta and Gamma Functions
6.1 Beta Function6.2 Properties of Beta Function6.3 Gamma Function6.4 Properties of Gamma Function6.5 Relation Between Beta and Gamma Functions6.6 Dirichlet's and Liouville's TheoremsExercises
7. Reduction Formulas
7.1 Reduction Formulas for ∫sinn xdx and ∫cosn xdx7.2 Reduction Formulas for ∫sinm x cosn x dx7.3 Reduction Formulas for ∫tann xdx and ∫secn xdx7.4 Reduction Formulas for ∫xn and ∫xn cos mxdx7.5 Reduction Formulas for ∫ xn eax dx and ∫ xm (log x)n dx7.6 Reduction Formula for ∫cosm x sin nxdxExercises
8. Volumes and Surfaces of Solids of Revolution
8.1 Volume of the solid of Revolution (Cartesian Equations)8.2 Volume of the Solid of Revolution (Parametric Equations)8.3 Volume of the Solid of Revolution (Polar Curves)8.4 Surface of the Solid of Revolution (Cartesian Equations)8.5 Surface of the Solid of Revolution (Parametric Equations)8.6 Surface of the Solid of Revolution (Polar Curves)Exercises
9. Multiple Integrals
9.1 Double Integrals9.2 Properties of a Double Integral9.3 Evaluation of Double Integrals (Cartesian Coordinates)9.4 Evaluation of Double Integrals (Polar Coordinates)9.5 Change of Variables in a Double Integral9.6 Change of Order of Integration9.7 Area Enclosed by Plane Curves (Cartesian and Polar Coordinates)9.8 Volume and Surface Area as Double Integrals9.9 Triple Integrals and their Evaluation9.10 Change to Spherical Polar Coordinates from Cartesian Coordinates in a Triple Integral9.11 Volume as a Triple IntegralExercises
10. Vector Calculus
10.1 Differentiation of a Vector10.2 Partial Derivatives of a Vector Function10.3 Gradient of a Scalar Field10.4 Geometrical Interpretation of a Gradient10.5 Properties of a Gradient10.6 Directional Derivatives10.7 Divergence of a Vector-Point Function10.8 Physical Interpretation of Divergence10.9 Curl of a Vector-Point Function10.10 Physical Interpretation of Curl10.11 The Laplacian Operator ∇210.12 Properties of Divergence and Curl10.13 Integration of Vector Functions10.14 Line Integral10.15 Work Done by a Force10.16 Surface Integral10.17 Volume Integral10.18 Gauss's Divergence Theorem10.19 Green's Theorem in a Plane10.20 Stoke's Theorem
Exercises
11. Three-Dimensional Geometry
11.1 Coordinate Planes11.2 Distance Between Two Points11.3 Direction Ratios and Direction Cosines of a Line11.4 Section Formulae—Internal division of a line by a point on the line11.5 Straight Line in Three Dimensions11.6 Angle Between Two Lines11.7 Shortest Distance Between Two Skew Lines11.8 Equation of a Plane11.9 Equation of a Plane Passing Through a Given Point and Perpendicular to a Given Direction11.10 Equation of a Plane Passing Through Three Points11.11 Equation of a Plane Passing Through a Point and Parallel to Two Given Vectors11.12 Equation of a Plane Passing Through Two Points and Parallel to a Line11.13 Angle Between Two Planes11.14 Angle Between a Line and a Plane11.15 Perpendicular Distance of a Point From a Plane11.16 Planes Bisecting the Angles Between Two Planes11.17 Intersection of Planes11.18 Planes Passing Through the Intersection of Two Given Planes11.19 Sphere11.20 Equation of a Sphere Whose Diameter is the Line Joining Two Given Points
11.21 Equation of a Sphere Passing Through Four Points
11.22 Equation of the Tangent Plane to a Sphere11.23 Condition of Tangency11.24 Angle of Intersection of Two Spheres11.25 Condition of Orthogonality of Two Spheres11.26 Cylinder11.27 Equation of a Cylinder with given Axis and Guiding Curves11.28 Right Circular Cylinder11.29 Cone11.30 Equation of a Cone with Vertex at the Origin11.31 Equation of a Cone with Given Vertex and Guiding Curve11.32 Right Circular Cone11.33 Right Circular Cone with Vertex (α, β, γ), Semi Vertical Angle θ, and (l, m, n) the Direction Cosines of the Axis11.34 Conicoids11.35 Shape of an Ellipsoid11.36 Shape of the Hyperboloid of One Sheet11.37 Shape of the Hyperboloid of Two Sheets11.38 Shape of the Elliptic Cone11.39 Intersection of a Conicoid and a Line11.40 Tangent Plane at a Point of Central Conicoid11.41 Condition of Tangency11.42 Equation of Normal to the Central Conicoid at Any Point (α, β, γ) On ItExercises
Part II
12. Preliminaries
12.1 Sets and Functions12.2 Continuous and Piecewise Continuous Functions12.3 Derivability of a Function and Piecewise Smooth Functions12.4 The Riemann Integral12.5 The Causal and Null Functions12.6 Functions of Exponential Order12.7 Periodic Functions12.8 Even and Odd Functions12.9 Sequence and Series12.10 Series of Functions12.11 Partial Fraction Expansion of a Rational Function12.12 Special Functions12.13 The Integral Transforms
13. Linear Algebra
13.1 Concepts of Group, Ring, and Field13.2 Vector Space13.3 Linear Transformation13.4 Linear Algebra13.5 Rank and Nullity of a Linear Transformation13.6 Matrix of a Linear Transformation13.7 Normed Linear Space13.8 Inner Product Space13.9 Matrices13.10 Algebra of Matrices13.11 Multiplication of Matrices13.12 Associtative Law for Matrix Multiplication13.13 Distributive Law for Matrix Multiplication13.14 Transpose of a Matrix13.15 Symmetric, Skew-symmetric, and Hermitian Matrices13.16 Lower and Upper Triangular Matrices13.17 Adjoint of a Matrix13.18 The Inverse of a Matrix13.19 Methods of Computing Inverse of a Matrix
13.20 Rank of a Matrix
13.21 Elementary Matrices13.22 Equivalence of Matrices13.23 Row and Column Equivalence of Matrices13.24 Row Rank and Column Rank of a Matrix13.25 Solution of System of Linear Equations13.26 Solution of Non-homogeneous Linear System of Equations13.27 Consistency Theorem13.28 Homogeneous Linear Equations13.29 Characteristic Roots and Vectors13.30 The Cayley-Hamilton Theorem13.31 Algebraic and Geometric Multiplicity of an Eigenvalue13.32 Minimal Polynomial of a Matrix13.33 Orthogonal, Normal, and Unitary Matrices13.34 Similarity of Matrices13.35 Triangularization of an Arbitrary Matrix13.36 Quadratic Forms
13.37 Diagonalization of Quadratic Forms
Exercises
14. Functions of Complex Variables
14.1 Basic Concepts14.2 Analytic Functions14.3 Integration of Complex-Valued Functions14.4 Power Series Representation of an Analytic Function14.5 Zeros and Poles14.6 Residues and Cauchy's Residue Theorem
14.7 Evaluation of Real Definite Integrals
14.8 Conformal MappingExercises
15. Differential Equations
15.1 Definitions and Examples15.2 Formulation of Differential Equation15.3 Solution of Differential Equation15.4 Differential Equations of First order15.5 Separable Equations15.6 Homogeneous Equations15.7 Equations Reducible to Homogeneous Form15.8 Linear Differential Equations15.9 Equations Reducible to Linear Differential Equations15.10 Exact Differential Equation15.11 The Solution of Exact Differential Equation15.12 Equations Reducible to Exact Equation15.13 Applications of First Order and First Degree Equations15.14 Linear Differential Equations15.15 Solution of Homogeneous Linear Differential Equation with Constant Coefficients
15.16 Complete Solution of Linear Differential Equation with Constant Coefficients
15.17 Method of Variation of Parameters to Find Particular Integral15.18 Differential Equations with Variable Coefficients15.19 Simultaneous Linear Differential Equations with Constant Coefficients15.20 Applications of Linear Differential Equations15.21 Mass-Spring System15.22 Simple Pendulum15.23 Solution in Series15.24 Bessel's Equation and Bessel's Function
15.25 Legendre's Equation and Legendre's Polynomial
15.26 Fourier–Legendre Expansion of a FunctionExercises
16. Partial Differential Equations
16.1 Formulation of Partial Differential Equation16.2 Solutions of a Partial Differential Equation16.3 Non-linear Partial Differential Equations of the First Order16.4 Charpit's Method16.5 Some Standard forms of Non-linear Equations16.6 The Method of Separation of Variables16.7 One-Dimensional Heat Equation16.8 One-DimensionalWave Equation16.9 Two-Dimensional Heat Equation
Exercises
17. Fourier Series
17.1 Trigonometric Series17.2 Fourier (or Euler) Formulae17.3 Periodic Extension of a Function17.4 Fourier Cosine and Sine Series17.5 Complex Fourier Series17.6 Spectrum of Periodic Functions17.7 Properties of Fourier Coefficients17.8 Dirichlet's Kernel17.9 Integral Expression for Partial Sums of a Fourier Series17.10 Fundamental Theorem (Convergence Theorem) of Fourier Series17.11 Applications of Fundamental Theorem of Fourier Series17.12 Convolution Theorem for Fourier Series17.13 Integration of Fourier Series17.14 Differentiation of Fourier Series17.15 Examples of Expansions of Functions in Fourier Series17.16 Signals and Systems17.17 Classification of Signals17.18 Classification of Systems17.19 Response of a Stable Linear Time Invariant Continuous Time System (LTC System) to a Piecewise Smooth and Periodic Input17.20 Application to Differential Equations17.21 Application to Partial Differential EquationsExercises
18. Fourier Transform
18.1 Fourier Integral Theorem18.2 Fourier Transforms18.3 Fourier Cosine and Sine Transforms18.4 Properties of Fourier Transforms18.5 Solved Examples18.6 Complex Fourier Transforms18.7 Convolution Theorem18.8 Parseval's Identities18.9 Fourier Integral Representation of a Function18.10 Finite Fourier Transforms18.11 Applications of Fourier Transforms18.12 Application to Differential Equations18.13 Application to Partial Differential EquationsExercises
19. Discrete Fourier Transform
19.1 Approximation of Fourier Coefficients of a Periodic Function19.2 Definition and Examples of DFT19.3 Inverse DFT19.4 Properties of DFT19.5 Cyclical Convolution and Convolution Theorem for DFT19.6 Parseval's Theorem for the DFT19.7 Matrix form of the DFT19.8 N-Point Inverse DFT19.9 Fast Fourier Transform (FFT)Exercises
20. Laplace Transform
20.1 Definition and Examples of Laplace Transform20.2 Properties of Laplace Transforms20.3 Limiting TheoremsExercises
21. Inverse Laplace Transform
21.1 Definition and Examples of Inverse Laplace Transform21.2 Properties of Inverse Laplace Transform21.3 Partial Fractions Method to Find Inverse Laplace Transform21.4 Heaviside's Expansion Theorem21.5 Series Method to Determine Inverse Laplace Transform21.6 Convolution Theorem21.7 Complex Inversion FormulaExercises
22. Applications of Laplace Transform
22.1 Ordinary Differential Equations22.2 Simultaneous Differential Equations22.3 Difference Equations22.4 Integral Equations22.5 Integro-Differential Equations22.6 Solution of Partial Differential Equation22.7 Evaluation of IntegralsExercises
23. The z-transform
23.1 Some Elementary Concepts23.2 Definition of z-transform23.3 Convergence of z-transform23.4 Examples of z-transform23.5 Properties of the z-transform23.6 Inverse z-transform23.7 Convolution Theorem23.8 The Transfer Function (or System Function)23.9 Systems Described by Difference EquationsExercises
24. Elements of Statistics and Probability
24.1 Measures of Central Tendency24.2 Measures of Variability (Dispersion)24.3 Measure of Skewness24.4 Measures of Kurtosis24.5 Covariance24.6 Correlation and Coefficient of Correlation24.7 Regression24.8 Angle Between the Regression Lines24.9 Probability24.10 Conditional Probability24.11 Independent Events24.12 Probability Distribution24.13 Mean and Variance of a Random Variable24.14 Binomial Distribution24.15 Pearson's Constants for Binomial Distribution24.16 Poisson Distribution24.17 Constants of the PoissonDistribution24.18 Normal Distribution24.19 Characteristics of the Normal Distribution24.20 Normal Probability Integral24.21 Areas Under the Standard Normal Curve24.22 Fitting of Normal Distribution to a Given Data24.23 Sampling24.24 Level of Significance and Critical Region24.25 Test of Significance for Large Samples24.26 Confidence Interval for the Mean
24.27 Test of significance for Single Proportion
24.28 Test of Significance for Difference of Proportion24.29 Test of Significance for Difference of Means24.30 Test of Significance for the Difference of Standard Deviations24.31 Sampling with Small Samples24.32 Significance Test of Difference Between Sample Means24.33 Chi-square Distribution24.34 χ2-test as a Test of Goodness-of-Fit24.35 Snedecor's F-Distribution24.36 Fisher's Z-DistributionExercises
25. Linear Programming
25.1 Linear Programming Problems25.2 Formulation of a Linear Programming Problem (LPP)25.3 Graphical Method to Solve Linear Programming Problem25.4 Canonical and Standard forms of Linear Programming Problem25.5 Basic Feasible Solution of an LPP25.6 Simplex Method25.7 Tabular form of the Solution25.8 Generalization of Simplex Algorithm25.9 Two-Phase Method25.10 Duality Property25.11 Dual Simplex Method25.12 Transportation Problems25.13 Matrix form of the Transportation Problem
25.14 Transportation Problem Table
25.15 Basic Initial Feasible Solution of Transportation Problem25.16 Test for the Optimality of Basic Feasible Solution25.17 Degeneracy in Transportation Problem25.18 Unbalanced Transportation ProblemsExercises
26. Basic Numerical Methods
26.1 Approximate Numbers and Significant Figures26.2 Classical Theorems Used in Numerical Methods26.3 Types of Errors26.4 General Formula for Errors26.5 Solution of Non-Linear Equations26.6 Linear System of Equations26.7 Finite Differences26.8 Error Propagation26.9 Interpolation
26.10 Interpolation With Unequal Spaced Points
26.11 Newton's Fundamental (Divided Difference) Formula26.12 Lagrange's Interpolation Formula26.13 Curve Fitting26.14 Numerical Quadrature (Integration)26.15 Ordinary Differential Equations26.16 Numerical Solution of Partial Differential EquationsExercises
Bibliography
Acknowledgements
Copyright

Content preview from Engineering Mathematics

Case III. Conjugate Complex Roots

Suppose that the auxiliary equation has a nonrepeated complex root α + iβ. Then, since the coefficients are real, the conjugate complex number α – iβ is also a non-repeated root. Thus, the solution given in (79) becomes

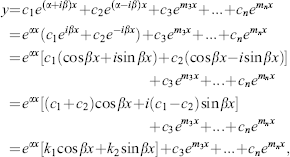
where k₁ = c₁ + c₂, k₂ = i(c₁ – c₂).
If two pairs of imaginary roots are equal, then

m₁ = m₂ = a + iβ and m³ = m⁴ = α – iβ.

Using Case II, the complete solution is

EXAMPLE 15.83

Solve

Solution. The symbolic form of the given equation is

(D³ + D² + 4D + 4)y = 0.

Therefore ...

Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
and much more.

Read now

Unlock full access

More than 5,000 organizations count on O’Reilly

O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.

Julian F.

Head of Cybersecurity

I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.

Addison B.

Field Engineer

I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.

Amir M.

Data Platform Tech Lead

I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.

Mark W.

Embedded Software Engineer

Publisher Resources

ISBN: 9789332515611

Cloud Computing

Data Engineering

Data Science

AI & ML

Programming Languages

Software Architecture

IT/Ops

Security

Design

Business

Soft Skills