Handbook of Compressible Aerodynamics

Chapter 6

Dimensionless Equations: Similarity Parameters

6.1. Normalization of equations: Reynolds, Froude, and Prandtl numbers

We consider the following basic equations, written in tensorial form:

– continuity equation:

– equation of motion:

The last term of this equation represents the body forces acting within the fluid. In what follows, we consider this to be gravity whose expression is:

ρf_i = ρge_i

where g is the gravity acceleration and e_i the unit vector indicating the direction of its action, generally the local vertical.

– energy equation:

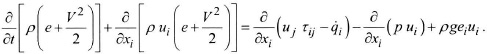
We assume that the gas is calorically perfect and the stress tensor τ_ij can be written as:

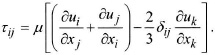
The purpose here is to give these equations dimensionless form by introducing non-dimensional quantities. This normalization operation is intended, in particular, to evaluate the relative importance of terms occurring in these equations that can lead to simpler forms if certain terms are very small, and therefore negligible, compared to other terms. Also, normalization will allow us to define similarity rules that enable us to transpose the obtained ...