Hands-On Mathematics for Deep Learning

The multivariate normal theory

In this section, we have so far dealt with random variables or a vector of iid random variables. Now, let's suppose we have a random vector, 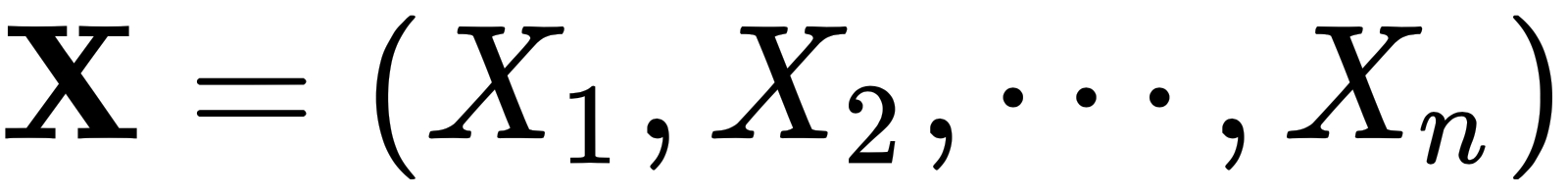, where the X_i values are all correlated.

Now, if we want to find the mean of X, we do so as follows:

If it exists, the covariance matrix is as follows:

Additionally, if we have , then and .

If we're dealing with two random vectors, then we have the following:

Now, let's ...