Introduction to the Variational Formulation in Mechanics

CFunctionals and the Gâteaux Derivative

C.1 Introduction

In this appendix we study in more detail several results that were utilized in Chapter 4 when dealing with the stiffness mechanical operators, with the Gâteaux derivatives of functionals, and with convex functions.

C.2 Properties of Operator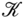

Let be the deformation rate operator satisfying the properties already established in Chapter 3 and let be the stiffness operator corresponding to the analyzed hyperelastic materials (recall that is a positive definite symmetric fourth‐order tensor in the space of deformations). Next, we show a series of fundamental results.

The first result establishes that

(C.1)

To prove (C.1) let us recall that is positive definite, and then it follows that , , so , . Therefore, we conclude that ,