Chapter 2Past, present, and future
2.1 Markov property and its extensions
2.1.1 Past 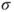 -field, filtration, and translation operators
-field, filtration, and translation operators
Let ![]() be a sequence of random variables. The
be a sequence of random variables. The ![]() -fields
-fields
contain all events that can be observed using exclusively ![]() , that is, the past up to time
, that is, the past up to time ![]() included of the sequence. Obviously
included of the sequence. Obviously
A family of nondecreasing ![]() -fields, such as
-fields, such as ![]() , is called a filtration, and provides a mathematical framework for the accumulation of information obtained by the step-by-step observation of the sequence.
, is called a filtration, and provides a mathematical framework for the accumulation of information obtained by the step-by-step observation of the sequence.
Product -field
Definition 1.2.1 gives an expression for the probability of any event ...
Get Markov Chains: Analytic and Monte Carlo Computations now with the O’Reilly learning platform.
O’Reilly members experience books, live events, courses curated by job role, and more from O’Reilly and nearly 200 top publishers.

