Mathematical Modeling in the Social and Life Sciences

APPENDIX III

Solving Systems of Equations

The equation

is an example of a linear equation in two unknowns x₁ and x₂. This equation is true for some values of the unknowns (for example, x₁ = 1, x₂ = − 1), but false for other values (for example, x₁ = 2, x₂ = 1). The set of all vectors

for which the equation is true is called the solution set of the equation. Any element of this set is called a solution of the equation.

It is easy to check that every solution of Eq. (1) is a vector of the form

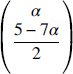
where α can be any real number. Conversely, every vector of this form is a solution of Eq. (1).

The general linear equation in two unknowns has the form

where a₁, a₂, and b are given constants and a₁ and a₂ are not both zero.

The general linear equation in n unknowns x₁, x₂, ..., x_n, is an equation of the form

where the a₁, a₂, ..., a_n, and b are given constants and at least one of the a_i's is nonzero. The set of all vectors

for which Eq. (3) is true is called the solution set of (3).

Since a linear ...