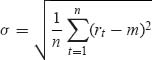Mathematics of the Financial Markets: Financial Instruments and Derivatives Modelling, Valuation and Risk Issues

12

Volatility and volatility derivatives

This chapter may be viewed as a continuation of Chapter 10. As seen in Chapter 10, Section 10.1, the volatility, denoted σ, originates from processes such as the general Wiener process used to model an underlying, and appears as a key ingredient for pricing non-conditional derivatives such as options. As such, strictly speaking, options should be only used to take (or to hedge) a position on the volatility. In Chapter 10, Section 10.1, we have made the distinction between the historical volatility, that is, the standard deviation of (past) prices changes, and the implied volatility used to price an option, as a guess at what level the volatility will happen to be during the lifetime of the option. Ex post, we may compare the implied volatility used for option pricing, and the corresponding actual historical volatility on the same period (see below). On an ex post basis, the actual historical volatility is understandably also called “realized volatility”, but this expression is not used here, to avoid confusion with the so-called “realized volatility” models, as presented in Section 12.3.

Strictly speaking, for a statistician, the usual formula for computing a standard deviation, hence the historical volatility, on a full1 population of n returns rt with a mean m, is actually the “standard estimator” of this standard deviation:

(12.1)

It is ...