Measure, Probability, and Mathematical Finance: A Problem-Oriented Approach

CHAPTER 28

BROWNIAN MOTION

Brownian motion is a fundamentally important stochastic process in that it is a central notion throughout the theoretical development of stochastic processes. In this chapter, we present the definition and some properties of Brownian motion.

28.1 Basic Concepts and Facts

Definition 28.1 (Standard Brownian Motion). Let (Ω, , P) be a probability space. A stochastic process {B_t : t ≥ 0} on (Ω, , P) is called a standard Brownian motion if it satisfies the following conditions:

(a) P{B₀ = 0} = P{ω : B₀(ω) = 0} = 1.

(b) For any 0 ≤ s < t, the increment B_t − B_s is a random variable normally distributed with mean 0 and variance t − s, that is, for any a < b, we have

(c) B_t has independent increments, that is, for any 0 ≤ t₁ < t₂ < … < t_n, the random variables B_t1, B_t2 − B_t1,…, B_tn − B_tn−1 are independent.

(d) Almost all sample paths of B_t are continuous functions:

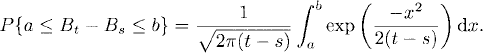
Definition 28.2 (Brownian Motion with Respect to Filtrations). Let {_t : t ≥ 0} be a filtration. A stochastic ...