Reliability Engineering

Appendix E:Percentage Points 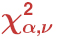of the Chi-Square Distribution

Let X₁, … , X_n be a random sample from a normal distribution with mean μ and variance σ², and let S² be the sample variance. Then the random variable

has a chi-square (χ²) distribution with n − 1 degrees of freedom.

The probability density function of a χ² random variable is

where k is the number of degrees of freedom. The mean and variance of the χ² distribution are k and 2k, respectively. The limiting form of χ² distribution as k → ∞ is the normal distribution.

The percentage points of the χ² distribution are given in the following table. We define as the percent point or value of the chi-square random variable with ν degrees of freedom such that the probability that χ² exceeds this value is α. We can write it as

The χ² distribution is skewed, and hence we need to find separate value for from the table.