Semi-Riemannian Geometry

Chapter 17 Smooth Manifolds with Boundary

It is not unusual in practice to encounter what would otherwise be a smooth manifold except for the presence of some type of “boundary” In this chapter, we introduce smooth manifolds with boundary and prove one of most important results in differential geometry—Stokes's theorem.

17.1 Smooth Manifolds with Boundary

The closed upper half‐space of ℝ ^m , defined by

is the model for what we later call a smooth m‐manifold with boundary. It is easily shown that

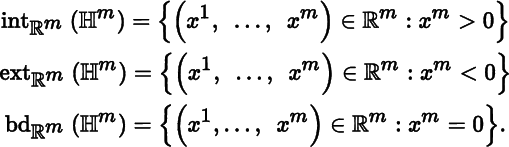
For example, ℍ³ = {(x,  y,  z) ∈ ℝ³ : z ≥ 0} is the upper half of ℝ³ including the xy‐plane, is the upper half of ℝ³ excluding the xy‐plane, is the lower half of ℝ³ excluding the xy‐plane, and is the xy‐plane.

Throughout, ℍ ^m is assumed to have the subspace topology induced by ℝ ^m .

Our first goal is to use ℍ ^m to broaden our earlier notion of “chart” Let M be a topological space, and consider the following modification of the definition of chart given in ...