March 2003
Intermediate to advanced
1056 pages
32h 58m
English
book
Transport Processes and Separation Process Principles (Includes Unit Operations) Fourth Edition
by Christie John Geankoplis
Content preview from Transport Processes and Separation Process Principles (Includes Unit Operations) Fourth EditionBecome an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.
3.7. DIFFERENTIAL EQUATIONS OF MOMENTUM TRANSFER OR MOTION
3.7A. Derivation of Equations of Momentum Transfer
The equation of motion is really the conservation-of-momentum equation (2.8-3), which we can write as
Equation 3.7-1
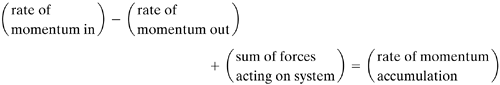
We will make a balance on an element as in Fig. 3.6-2. First we shall consider only the x component of each term in Eq. (3.6-30). The y and z components can be described in an analogous manner.
The rate at which the x component of momentum enters the face at x in the x direction by convection is (ρνxνx)x Δy Δz, and the rate at which it leaves at x + Δx is (ρνxνx)x+Δx Δy Δz. The quantity (ρvx) is the concentration in momentum/m ...
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
and much more.
Read now
Unlock full access
More than 5,000 organizations count on O’Reilly
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.Julian F.
I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.Addison B.
I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.Amir M.
I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.