Cost of Capital: Applications and Examples, + Website, 5th Edition

APPENDIX 11B

Estimating Beta: Interpreting Regression Statistics

Mark W. Shirley and Niel Patel

Introduction

Evaluating Beta Estimation Output

Evaluating Regression Output

Regression Statistics Table

ANOVA Table

Regression Coefficient Table

Beta Estimation—TIBCO Software, Inc. (TIBX) Example

INTRODUCTION

As discussed in Chapter 11 for a publicly traded stock, beta is often estimated by regression analysis (ordinary least squares [OLS] regression). In applying regression analysis to beta estimation, excess returns on the individual security R_i – R_f are regressed against the excess returns on the market R_m – R_f during a look-back period. The look-back period is the historic period of sufficient time series to include data reflective of changes in macroeconomic conditions. The resulting slope of the best-fit line is the beta estimate. Formula 11.1 repeated here as Formula 11B.1 illustrates the regression formula:

(Formula 11B.1)

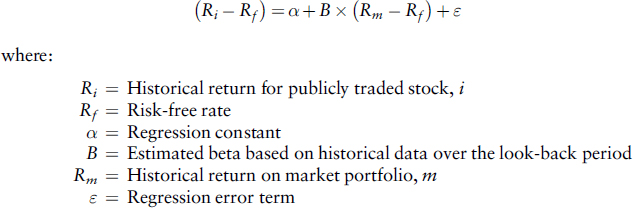
The Greek letters β and α are explained in Appendix V, Review of Statistical Analysis and in the discussions of hypothetical testing and types of error.¹ These symbols have different meanings when used in the context of regression analysis. However, error measurement (residuals) is a fundamental and critical analysis in determining the reliability of a regression line.

Comparisons of beta estimates based on excess returns or total returns, as a practical ...