October 2006
Intermediate to advanced
369 pages
8h 11m
English
Content preview from Digital Filters Design for Signal and Image Processing
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.
4.2. Different types of filters and filter specifications
Let us consider the example of an ideal low-pass filter of normalized gain and whose frequency is in relation to cut-off frequency (see Figure 4.1).
With an ideal filter, transmission is total in the passband and the stopband.
We write x as the normalized frequency in relation to the cut-off frequency:
NOTE.– x is also called the normalized angular frequency in relation to the cut-off angular frequency: 
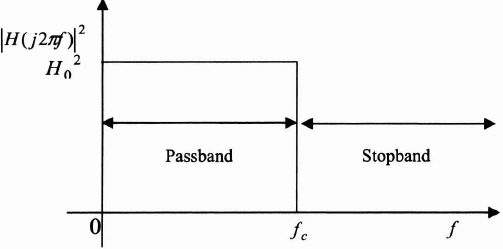
Figure 4.1. Ideal low-pass filter
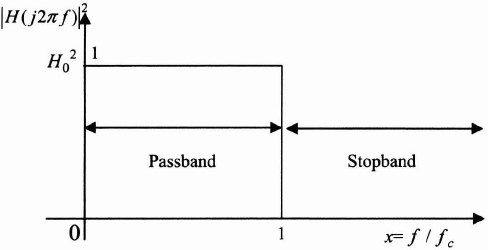
Figure 4.2. Low-pass filter corresponding to Figure 4.1, normalized in frequency and amplitude
In general, we will deduce normalized high-pass, band-pass and band-stop filters from normalized low-pass filters by applying frequency variable change formulae (see Figures 4.3, 4.4 and 4.5).
Obtaining the transfer function of the filter H(j2πf) from the transfer function of the normalized low-pass filter H(jx) follows the frequency transformation summarized in Table 4.1.
| Obtaining a filter | Transformation carried out from the transfer function of the normalized low-pass filter |
| High-pass with ... |
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
and much more.
Read now
Unlock full access
More than 5,000 organizations count on O’Reilly
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.Julian F.
I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.Addison B.
I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.Amir M.
I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.