1.5. Solving the electromagnetic and thermal equations
The electromagnetic and thermal equations are partial differential equations, where the unknown is either a scalar or a vector quantity dependent on spatial coordinates and time. Numerical methods are often used to solve these equations. Nevertheless, in certain simple cases and for the comprehension of the involved physical phenomena, analytic methods are interesting.
1.5.1. Analytic methods
1.5.1.1. Transient state
In the one-dimensional case, the general form of the electromagnetic and thermal equations is as follows:
[1.58] 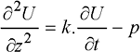
where, z is the propagation direction of the wave U and, p, represents internal sources.
For example, the one-dimensional formulation of magnetic potential A is:
[1.59] 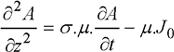
and for the formulation in H, we obtain:
[1.60] 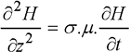
As for the thermal equation, it can be written as:
[1.61] 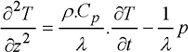
1.5.1.2. Harmonic state
For a sinusoidal variation, the general form is given by:
[1.62] 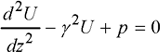
where with as the penetration depth of wave ...
Get Electrothermics now with the O’Reilly learning platform.
O’Reilly members experience books, live events, courses curated by job role, and more from O’Reilly and nearly 200 top publishers.

