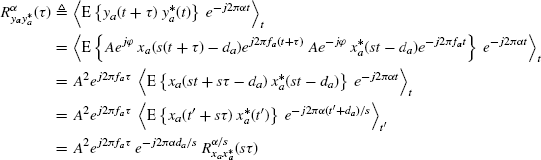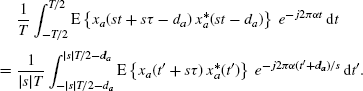Generalizations of Cyclostationary Signal Processing: Spectral Analysis and Applications

7.10 Proofs

7.10.1 Proof of (7.262)

Fourier transform of a time-scaled, delayed, and frequency-shifted signal:

(7.383)

(7.384)

(7.385)

7.10.2 Proof of (7.263) and (7.264)

By using (7.261), the result is that

(7.386)

where is the continuous-time infinite average with respect to t, and, in the third equality, the variable change t′ = st − d_a is made, so that

(7.387)

This proof can be equivalently carried out in the FOT approach by simply removing the expectation operator E{ · } in (7.386) and (7.387).

(7.264) is obtained by Fourier transforming both sides of (7.263).

7.10.3 Proof of (7.262) and (7.272)

By reasoning as for (7.386), we have

(7.388)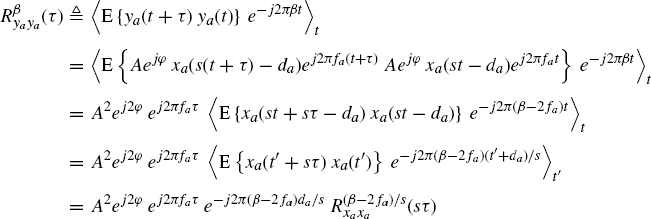

(7.268) is obtained by Fourier transforming both sides of (7.267).

7.10.4 Proof of (7.273)

Accounting for (7.262) and (7.272), one obtains

(7.389) ...