September 2012
Beginner to intermediate
536 pages
14h 40m
English
book
Loss Models: From Data to Decisions, 4th Edition
by Stuart A. Klugman, Harry H. Panjer, Gordon E. Willmot
Content preview from Loss Models: From Data to Decisions, 4th Edition
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.
19.5 Exercises
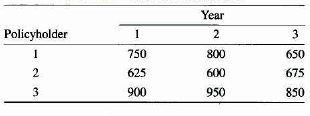
19.1 Past claims data on a portfolio of policyholders are given in Table 19.4. Estimate the Bühlmann credibility premium for each of the three policyholders for year 4.
Table 19.4 Data for Exercise 19.1.
19.2 Past data on a portfolio of group policyholders are given in Table 19.5. Estimate the Bühlmann–Straub credibility premiums to be charged to each group in year 4.
Table 19.5 Data for Exercise 19.2.
19.3 For the situation in Exercise 17.3, estimate the Bühlmann credibility premium for the next year for the policyholder.
19.4 Consider the Bühlmann model in Example 19.1.
(a) Prove that Var(Xij) = a + v.
(b) If {Xij : i = 1, …, r and j = 1, …, n} are unconditionally independent for all i and j, argue that an unbiased estimator of a + v is
(c) Prove the algebraic identity
(d) Show that, conditionally,
(e) Comment on the implications of (b) and (d).
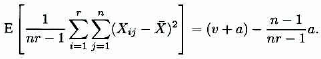
19.5 Suppose that the random variables Y1, …, Yn are independent with
Define b = b1 + b2 + ··· + bn and = ∑nj
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
and much more.
Read now
Unlock full access
More than 5,000 organizations count on O’Reilly
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.Julian F.
I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.Addison B.
I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.Amir M.
I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.