March 2018
Intermediate to advanced
864 pages
22h 49m
English
Content preview from Mathematical Methods in Science and Engineering, 2nd Edition
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.
Chapter 14Infinite Series
In physics and engineering, sometimes physical properties can only be expressed in terms of infinite sums. We also frequently encounter differential or integral equations that can only be solved by the method of infinite series. In working with infinite series, the first thing that needs to be checked is their convergence. In this regard, we start by introducing the commonly used tests of convergence for series of numbers and then extend our discussion to series of functions and power series. We then introduce some analytic techniques for evaluating infinite sums. We also discuss asymptotic series and the method of steepest descent and saddle-point integrals. We also introduce the Padé approximants, which is a very effective tool in finding sums of series whose only a few terms are known. Infinite products, which are closely related to infinite series, are also discussed in this chapter. In conjunction with the Casimir effect, we show how finite and meaningful results can be obtained from some of the divergent series in physics by the method of regularization and renormalization.
14.1 Convergence of Infinite Series
We write an infinite series with the general term 
as
14.1 
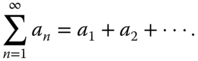
Summation of the first terms is called the th partial sum of the series. If the ...
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
and much more.
Read now
Unlock full access
More than 5,000 organizations count on O’Reilly
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.Julian F.
I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.Addison B.
I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.Amir M.
I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.