Modern Control System Theory and Design, 2nd Edition

PROBLEMS

7.1. Determine the circuit structure, the values of resistance and capitance, the gains of any amplifiers required, and the complex-plane plot for first-order networks having the following characteristics:

(a)  Phase lead of 60° at ω = 4 rad/sec, a minimum input impedance of 50,000 Ω, and an attentuation of 10 dB at dc.

(b)  Phase lag of 60° at ω = 4 rad/sec, a minimum input impedance of 50,000 Ω, and a high-frequency attenuation of 10 dB.

(c)  A phase-lag-lead network having an attenuation of 10 dB for a frequency range of ω = 1 to ω = 10 rad/sec and an input impedance of 50,000 Ω.

In all cases, limit the maximum values of resistance to 1 MΩ and capitance to 10 μF. Furthermore, assume that the loads on the networks have essentially infinite impedance.

7.2. The system illustrated in Figure P7.2 consists of a unity-feedback loop containing a minor-rate-feedback loop.

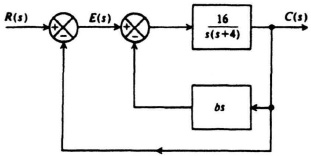
Figure P7.2

(a)  Without any rate feedback (b = 0), determine the damping ratio, undamped natural frequency, peak overshoot of the system to a unit step input, and the steady-state error resulting from a unit ramp input.

(b)  Determine the rate-feedback constant b which will increase the equivalent damping ratio of the system to 0.8.

(c)  With rate feedback and a damping ratio of 0.8, determine the maximum percent overshoot of the system to a unit step input and the steady-state error resulting from a unit ramp ...