Optical Communications, 2nd Edition

2.4 FOCUSING RANDOM FIELDS

Let us extend the concept of aperture-focal-plane focusing to the case of random arriving fields. This is of importance in communications since received optical noise is almost always present and, in many cases, the signal field itself may become random. Consider again the system in Figure 2.1, except assume that the received field f_r(t, r) is random. We know the resulting imaged field will also be random and is given by Eq. (2.1.11), which we rewrite as

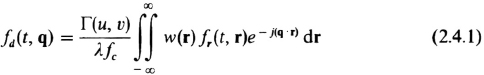
where r = (x, y) and q = (2π/λ)(u, ν), and w(r) represents the lens pupil function in Eq. (2.1.15),

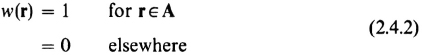
The random field f_d(t, q) will have a mutual coherence function

Since Γ(u, ν)Γ*(u, ν) = 1, this becomes

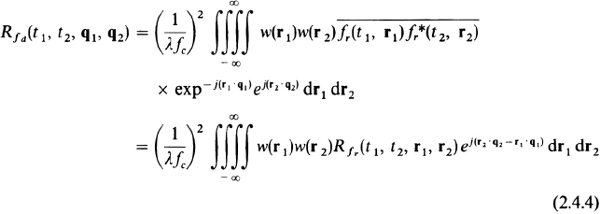
where R_fr denotes the mutual coherence function of the receiver field. Thus the received field coherence function is converted to the corresponding focal plane coherence function by Eq. (2.4.4).

Let us concentrate on the average field intensity at a time t and point q in the focal plane, defined by the irradiance

From Eq. (2.4.4),

If the received field is coherence separable and homogeneous,

and Eq. (2.4.6) ...