Signals and Systems

10.3 STATE MODEL OF A SECOND-ORDER SYSTEM

Let us consider the following equation for 2nd order system:

 

y(k + 2) + 4y(k + 1) + 3y(k) = u(k)     (10.6)

 

In Eq. (10.6) there are two delay elements i.e., y(k + 2) and y(k + 1). Figure 10.4 shows the way of obtaining y(k) from y(k + 2).

 

 

Fig. 10.4

 

From Eq. (10.6) we can write

 

y(k + 2) = u(k) − 3y(k) −4y(k + 1)     (10.7)

 

Let x₁(k) and x₂(k) be the output of delay elements of x₁(k + 1) and x₂(k + 1) respectively.

Figure. 10.5 shows the representation of Eq. (10.7).

 

 

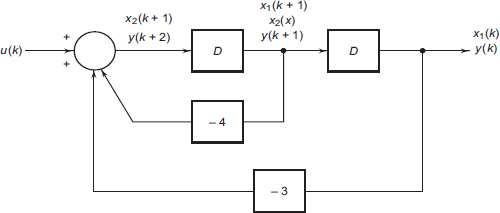
Fig. 10.5

 

From ...