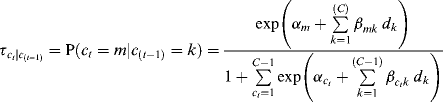Structural Equation Modeling: Applications Using Mplus

6.2 LTA model

The LTA model is a longitudinal mixture model for studying changes over time in classes or statuses of latent class variables (Graham et al., 1991; Collins and Wugalter, 1992; Lanza, Flaherty and Collins, 2003). Most often, LCA is utilized to identify the unobserved latent classes, statuses or states, using the same set of outcome measures at each time point. LTA can be considered an extension of LCA to longitudinal data, in which LCA plays the role of measurement model. Once latent classes are identified at different time points, a structural model is used to analyze transitions of latent class membership over time. Of course, the latent class classification and analysis on transitions of latent classes over time are conducted simultaneously in LTA, and interest focuses on transition between classes over time. Usually the first-order stationary transition is assumed so that class statuses at time t are only affected by statuses at time (t − 1) and this dependence is constant over time. The probability of transition to class m at time t from class k at (t − 1) is described in the following multinomial logistic regression of c_t on c_{(t − 1)} (Reboussin et al., 1998; Nylund, Asparouhov and Muthén, 2007):

(6.14)

where represents the probability of transition to latent class ...