Digital Filters Design for Signal and Image Processing

4.3. Butterworth filters and the maximally flat approximation

4.3.1. Maximally flat functions (MFM)

Here we look at a low-pass normalized transfer function whose squared amplitude is shown in equation (4.3). We try to find a filter with the flattest possible frequency response in the passband when x is close to 0. To come as close as possible to the specification, the synthesized filter must have an amplitude diagram as flat as possible when x = 0. For that, we find the conditions that allow us to cancel the successive derivations of the function |H(jx)|².

We know that the squared amplitude function |H(jx)|², being analytic when x=0, can be developed by using the McLaurin series as follows:

This development introduces the successive derivatives of |H(jx)|² used for x=0, or:

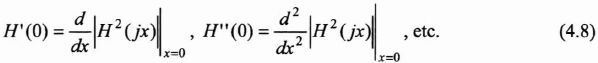
Moreover, |H(jx)|² being a rational fraction (see equation (4.3)), we can approach it by a polynomial by proceeding to the division following increasing powers of polynomials that constitute the numerator and the denominator of |H(jx)|². In this way we obtain the following development:

Since the McLaurin development is unique in the convergence region, we can then identify the coefficients of equations (4.7) and ...