Chapter 6. Operations on Distributions
Skewness
Skewness is a statistic that measures the asymmetry of a distribution. Given a sequence of values, xi, the sample skewness is:
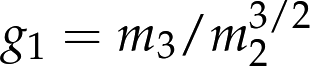
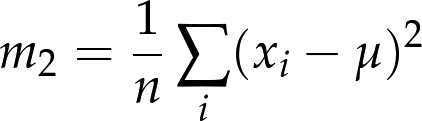
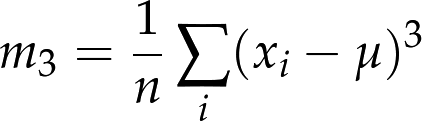
You might recognize m2 as the mean squared deviation (also known as variance); m3 is the mean cubed deviation.
Negative skewness indicates that a distribution “skews left”; that is, it extends farther to the left than the right. Positive skewness indicates that a distribution skews right.
In practice, computing the skewness of a sample is usually not a good idea. If there are any outliers, they have a disproportionate effect on g1.
Another way to evaluate the asymmetry of a distribution is to look at the relationship between the mean and median. Extreme values have more effect on the mean than the median, so in a distribution that skews left, the mean is less than the median.
Pearson’s median skewness coefficient is an alternative measure of skewness that explicitly captures the relationship between the mean, μ, and the median, μ1/2:
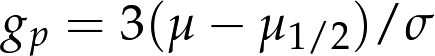
This statistic is robust, which means that it is less vulnerable to the effect of outliers. ...
Get Think Stats now with the O’Reilly learning platform.
O’Reilly members experience books, live events, courses curated by job role, and more from O’Reilly and nearly 200 top publishers.

