June 2016
Beginner
504 pages
14h 23m
English
Content preview from Understanding and Applying Basic Statistical Methods Using R
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.
Chapter 12MULTIPLE COMPARISONS
Chapter 10 described how to test the hypothesis that two or more independent groups have a common mean. In symbols, the goal was to test
But typically, one wants to know more about how the groups compare: which groups differ, how do they differ, and by how much?
Note that rather than test the hypothesis given by Equation (12.1), another approach is to test the hypothesis of equal means for all pairs of groups. For example, if there are four groups (
), methods in Chapter 9 could be used to test the hypothesis that the mean of the first group is equal to the mean of the second, the mean of first group is equal to the mean of third, and so on. In symbols, the goal is to test
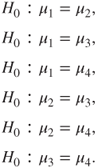
There is, however, a technical issue that needs to be taken into account. Suppose there are no differences among the groups in which case none of the six null hypotheses just listed should be rejected. To keep things simple for the moment, assume that all four groups have normal distributions with equal variances, in which case Student's 
test in Chapter 9 provides exact control ...
Become an O’Reilly member and get unlimited access to this title plus top books and audiobooks from O’Reilly and nearly 200 top publishers, thousands of courses curated by job role, 150+ live events each month,
and much more.
Read now
Unlock full access
More than 5,000 organizations count on O’Reilly
O’Reilly covers everything we've got, with content to help us build a world-class technology community, upgrade the capabilities and competencies of our teams, and improve overall team performance as well as their engagement.Julian F.
I wanted to learn C and C++, but it didn't click for me until I picked up an O'Reilly book. When I went on the O’Reilly platform, I was astonished to find all the books there, plus live events and sandboxes so you could play around with the technology.Addison B.
I’ve been on the O’Reilly platform for more than eight years. I use a couple of learning platforms, but I'm on O'Reilly more than anybody else. When you're there, you start learning. I'm never disappointed.Amir M.
I'm always learning. So when I got on to O'Reilly, I was like a kid in a candy store. There are playlists. There are answers. There's on-demand training. It's worth its weight in gold, in terms of what it allows me to do.