Digital Filters Design for Signal and Image Processing

2.6. Stability

The fact that the transfer function is a rational fraction naturally leads us to the issue of stability, which can be studied from considering the z-transform of the impulse response.

2.6.1. Bounded input, bounded output (BIBO) stability

A linear time invariant system is BIBO stable if its impulse response verifies the following relation (see also Chapter 10):

The transfer function is the z-transform of the impulse response; from there, we have, for all of z belonging to the ROC:

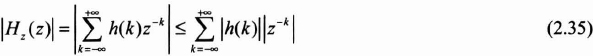
Now, on the unity circle in the complex plan z, we have:

From this the following result is obtained:

Many stability criteria have been developed to study the stability of filters. Among these, we first will look at the test of pole positions of the transfer function, then at Routh's and Jury's criteria.

2.6.2. Regions of convergence

In causal systems, a necessary and sufficient condition of stability is that all the poles of the transfer function must be inside the unity circle in the z-plane.

The decomposition of the basic elements of the transfer function of a discrete causal system H_z(z