Foundations of Electromagnetic Compatibility with Practical Applications

10Magnetically Coupled Circuits

10.1 Self and Mutual Inductance

Consider the circuit shown in Figure 10.1.

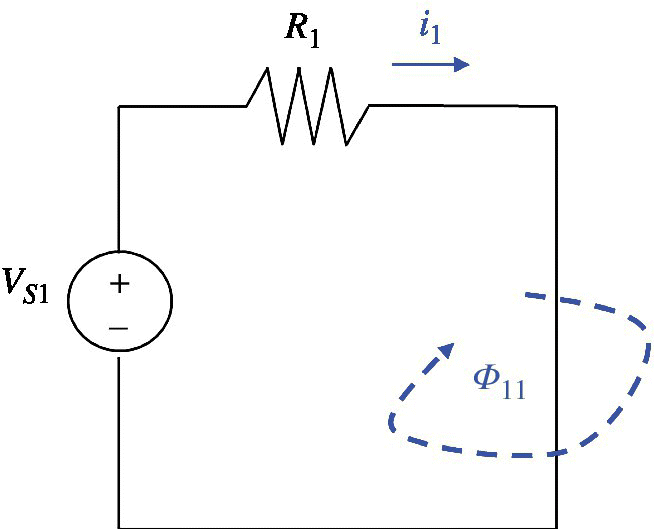
Figure 10.1 Magnetic flux produced by the current flowing in a loop.

Time‐varying current i₁(t) gives rise to the time‐varying flux Φ₁₁ that crosses the loop in which the current i₁ flows. According to Faraday’s law this time‐varying flux crossing the loop induces a voltage in the loop.

We model this by introducing the concept of the self inductance of circuit 1, defined as

(10.1)

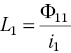
We then augment the circuit of Figure 10.1 to that shown in Figure 10.2.

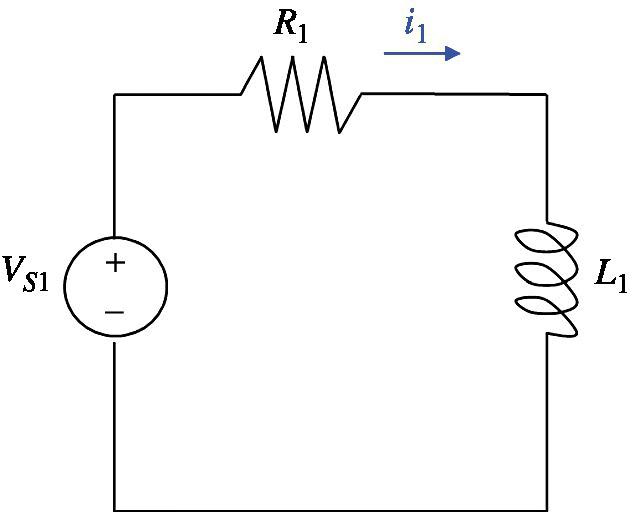
Figure 10.2 Loop self‐inductance.

Note that the loop inductance is a property of the loop itself and does not depend on the voltage V_S or the current i (just like the resistance of a resistor does not depend on the voltage across it or the current through it).

That is, any closed loop will have its own self inductance, whether current flows through it or not. It can, therefore, be represented by the circuit model shown in Figure 10.3.

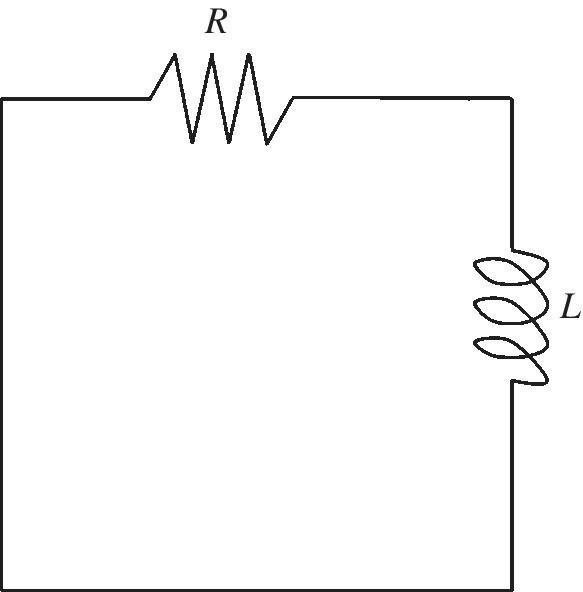
Figure 10.3 Circuit model of a loop.

Now consider the situation where another circuit (with its own self inductance) ...